Friction Damping 1
Energy Approach
In reality, unless oscillations are supported by some outside force, they gradually diminish in amplitude and, eventually, stop.
There are many reasons why it happens - some forces inside oscillating mechanism, like not ideal elasticity, external forces that prevent oscillation, like friction, viscosity of the medium surrounding the oscillating mechanism etc.
The oscillations that gradually diminish their amplitude because of one of above or similar factors are called damped oscillations.
The force that prevents the oscillations to continue indefinitely is called a damping force.
In this lecture we analyze the oscillation that experiences an external damping force of friction.
Our base oscillating mechanism is presented below. We will use it to demonstrate the damping effect of a friction against the horizontal surface, assuming our weightless spring conforms to the Hooke's Law and an object attached to it can be considered a point-mass.
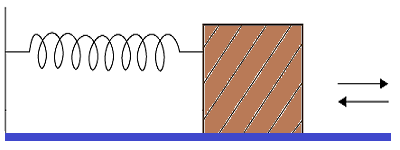
Let's assume that object of mass m oscillates horizontally along the X-axis on a spring with elasticity k, while lying on a horizontal surface in the gravitational field of the Earth with free fall acceleration g and, therefore, experiences the constant force of friction as it moves left and right on the spring.
In this case there is a constant friction force acting against the movement equaled to
Ff = μ·m·g
where μ is the coefficient of friction.
For simplicity, we will not differentiate friction at motion (kinetic friction) from friction at rest (static friction) and assume they are both equal to the expression above.
Let x(t) be a horizontal displacement of the object from its neutral position on a spring, where a spring is not stretched or squeezed. When this displacement is positive, a spring is stretched, when negative, a spring is squeezed.
As in previous lectures, our initial conditions on the position and speed of an object at time t=0=t0 are:
x(0) = a > 0 (initial stretched position of a spring with an object at distance a from the neutral position);
x'(0) = 0 (no initial speed, we just let the spring go by itself).
Since our ideal spring conforms to the Hooke's Law, which is symmetrical relative to stretching and squeezing a spring, we will examine in details only when a spring is initially stretched.
For oscillation to start, we have to assume that the force of a spring Fs stretched by an initial distance a (according to Hooke's Law, Fs=−k·a) is greater by absolute value than the friction force Ff=μ·m·g.
So, the necessary condition for a movement to start is
k·a > μ·m·g
or
a > μ·m·g/k = λ
where λ is a constant that combines the characteristics of a spring's elasticity k, object's mass m and friction μ·g, a compounded characteristic of an oscillating system as a whole.
Let's call this characteristic a system's critical distance.
Two points, x=λ and x=−λ, that are positioned exactly at critical distance from the neutral point x=0, we will call critical points with the former being a critical point of stretching and the latter - critical point of squeezing.
If, holding an object, we stretch a spring by distance a and then let it go, to start a movement, an object should be at a displacement from the neutral position greater by absolute value than critical distance λ. Otherwise, it will remain at rest, the pulling force of a spring would not be sufficient to overcome the friction force.
Let's assume that our initial spring stretch a is greater than critical distance λ, that is beyond the critical point of stretching. We expect that the pulling force of a spring will overcome friction, and an object will start moving in the negative direction of the X-axis towards the neutral position and, possibly, beyond it. As it moves, its potential energy is partially spent on friction.
Sooner or later it will stop, not reaching the point of x=−a on the opposite side from the neutral point, because, if it did, its potential energy would be the same as in the beginning of motion, which is impossible since energy is partially spent to do some work against the force of friction.
To find the stop point b, we will use the energy considerations.
When an object started its motion at time t0=0 at position x(t0)=a, its total (potential) energy, as we discussed in the previous lecture, was
U(t0) = k·a²/2
When, after moving in negative direction along X-axis, it stops at coordinate b (positive or negative) at some time t1, its total (potential) energy is
U(t1) = k·b²/2
Since constant friction force Ff=μ·m·g was acting all the time against the object's motion, from the Law of Energy Conservation follows that the work performed by this friction force during the time of motion W[a,b]=Ff·(a−b) equals to a difference in potential energies at the beginning and at the end of motion:
U(t0) − U(t1) = W[a,b]
From this equation follows:
k·a²/2 − k·b²/2 = μ·m·g·(a−b)
(a²−b²)/2 = μ·m·g·(a−b)/k
(a−b)·(a+b)/2 = λ·(a−b)
(a+b)/2 = λ
which means that the position at the critical distance from the neutral point, that is, critical point, is a midpoint between the beginning and the end of a motion.
This is a very important observation about the role of critical points. We came up with it for initial stretching of a spring. Because the Hooke's Law is symmetrical for stretching and squeezing, exactly the same formula is true when we initially squeeze a spring with the only difference that we will use the critical point on the squeezing side x=−λ as a point of symmetry.
In other words, if we stretch a spring beyond the critical point of stretching x=λ and let it go, the object will start moving to a negative direction along the X-axis and will stop at a point symmetrical to the starting point relatively to the critical point of stretching.
Analogously, if we squeeze a spring beyond the critical point of squeezing x=−λ and let it go, the object will start moving to a positive direction along the X-axis and will stop at a point symmetrical to the starting point relatively to the critical point of squeezing.
Back to initial stretching (a>0), we can resolve the last equation for b to find exact position where our object stops its motion
a + b = 2λ
b = 2λ − a = −(a − 2λ)
Let's analyze this formula for different values of initial stretch distance a>0.
1. If initial stretch a is less than critical distance λ, new position b=2λ−a will be greater than critical distance λ. That is, we stretch a spring, let it go, and it will stretch even further by itself, which is impossible. This confirms that the motion in this case is impossible, the object will stay at rest.
2. If λ<a≤2λ, the object stops at distance 0≤b<λ from the neutral point. It's not negative, which means that an object will not go beyond the neutral point. Also, it's within the critical distance from the neutral point, which means that after stop it will not move any further, the force of a spring will not overcome friction.
3. If 2λ<a≤3λ, the object stops at distance −λ≤b<0 from the neutral point. It's negative, which means that an object will go beyond the neutral point and will squeeze a spring. Also, it's within the critical distance from the neutral point on the negative (squeezing) side, which means that after stop it will not move any further, the force of a spring will not overcome friction.
4. If 3λ<a≤4λ, the object stops at distance −2≤b<−λ from the neutral point. It's negative, which means that an object will go beyond the neutral point and will squeeze a spring. Also, it's distance from the neutral point (on the squeezing side) is greater than the critical distance, the spring is squeezed beyond the critical point of squeezing, which means that the force of a spring is greater than friction and, after stop, an object will move in the opposite (positive) direction towards the neutral position.
At this point it makes sense to consider this situation as the new beginning, and use the initial position b=2λ−a instead of a, repeating all the calculations, coming with a point x=c, where our object stops the next time, that is symmetrical to x=b relatively to the critical point of squeezing x=−λ:
(b+c)/2 = −λ
c = −2λ − b
c = −2λ − (2λ−a) = a − 4λ
The last case demonstrates that the absolute value of deviation of an object from the neutral position, the amplitude of its oscillation, is diminishing by 2λ in each direction every time.


No comments:
Post a Comment