AM Equation: UNIZOR.COM - Physics4Teens - Waves - Radio

Notes to a video lecture on http://www.unizor.com

AM Equation

Let's approach amplitude modulation (AM) from a mathematical standpoint.

High frequency oscillations of the electric current in the LC circuit with an inductor of inductance L and a capacitor of capacitance C can be described by a function
I(t) = A_c·cos(ω_c·t)
where
I(t) is the circuit's own oscillation of the current,
A_c is the amplitude of these oscillations,
ω_c = 1/√L·C is an angular frequency of these oscillations.

Simple air pressure oscillations resulting from a sound can be expressed by a similar function P(t) = A_s·cos(ω_s·t)
where
P(t) is the oscillation of the air pressure around a source of sound,
A_s is the amplitude of these air pressure oscillations,
ω_s is an angular frequency of these oscillations.

Note that our design requires ω_c to be substantially greater than ω_s.
Also note that real sound is a combination (superposition) of different overtones with different amplitudes and different angular frequencies and phases, like
P(t) = A_s1·cos(ω_s1·(t+φ_s1)) +
+ A_s2·cos(ω_s2·(t+φ_s2)) +
+ A_s3·cos(ω_s3·(t+φ_s3)) + ...

Amplitude modulation alters the amplitude of the carrier's oscillations by changing it in synch with sound oscillations.
The simplest way to achieve it mathematically is to incorporate the sound waves into an amplitude of carrier's waves:
I_m(t) = [A_c+P(t)]·cos(ω_c·t)

Here is an example of this type of modulation of a carrier's signal.

Assume, the carrier's high frequency oscillations have an amplitude A_c=4 and angular frequency ω_c=20, which can be described by an equation
I(t) = 4·cos(20t)

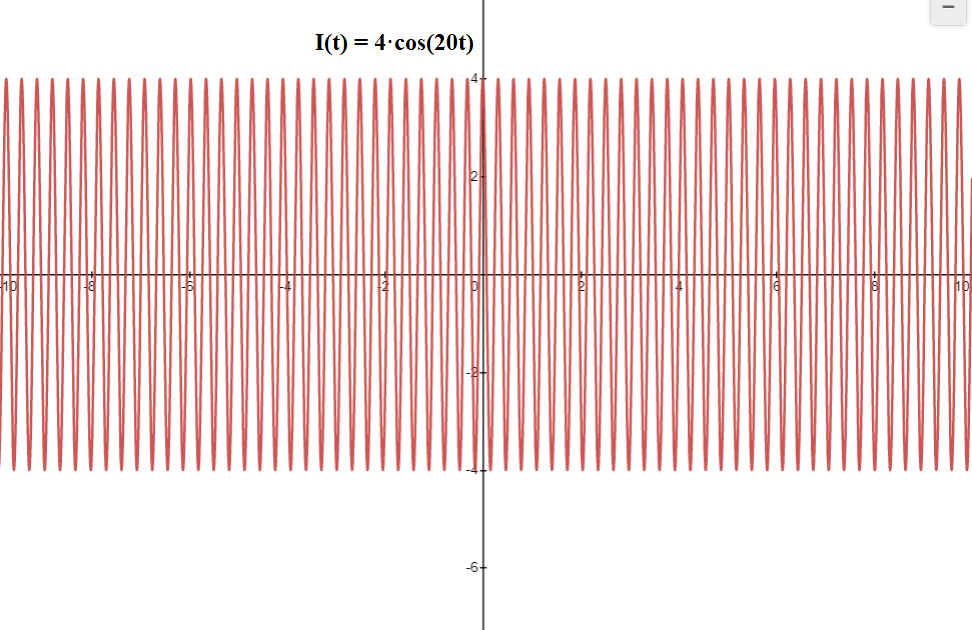
(you can click the right mouse button and open this picture in another tab for better view)

The sound makes air pressure oscillations that combine two different tones, one with an amplitude A_s1=2 and angular frequency ω_s1=1 and another with an amplitude A_s2=1 and angular frequency ω_s2=2, which can be described by the following equation
P(t) = 2·cos(t) + cos(2t)

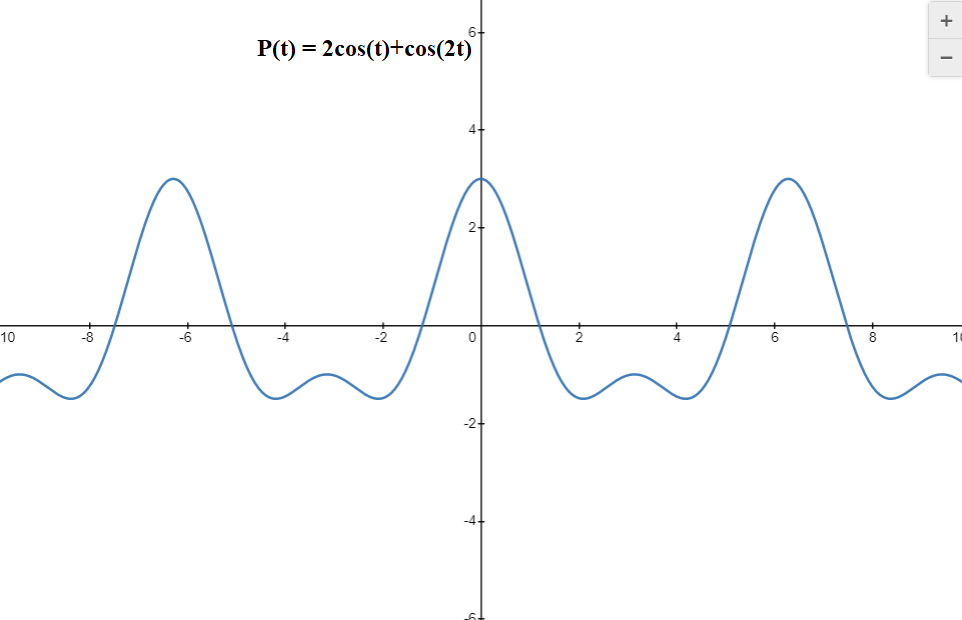
Then the modulated signal can be described by an equation
I_m(t) =
= [4+2·cos(t)+cos(2t)]·cos(20t)
that graphically looks like this:


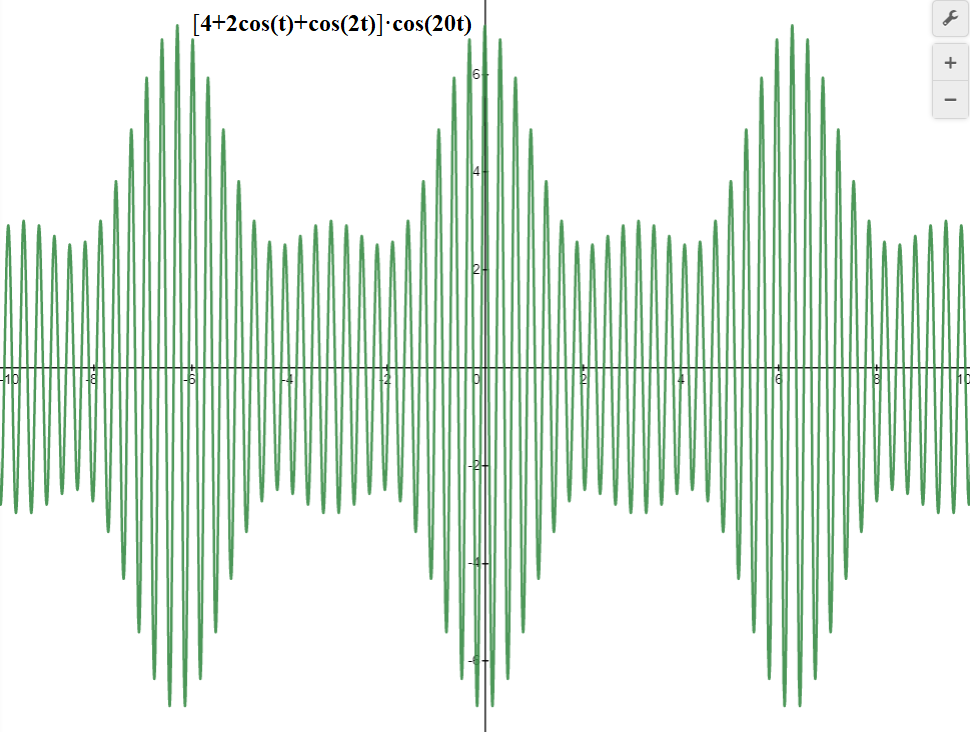
Just as a demonstration of the importance of having a high carrier frequency of a signal to properly represent a sound, here is what the transmitted signal would look like if the LC circuit of a carrier has a frequency comparable to a frequency of sound.

Assume, the carrier's oscillations have an amplitude A_c=4 and angular frequency ω_c=3, which can be described by an equation
I(t) = 4·cos(3t)
The sound waves are assumed to be as above
P(t) = 2·cos(t) + cos(2t)
Then the modulated signal can be described by an equation
I_m(t) =
= [4+2·cos(t)+cos(2t)]·cos(3t)
The following graph represents both the sound wave (purple) and modulated signal (blue):

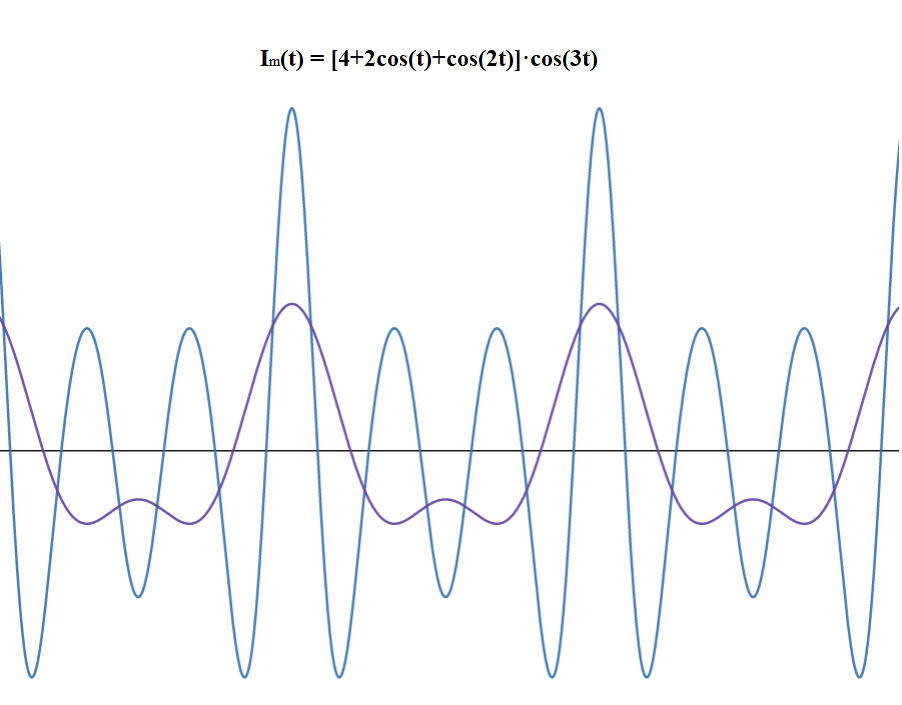
As you see, the representation of sound waves by a modulated signal is far from exact. This lower frequency of amplitude modulation cannot be used for transmitting sound.