Length Transformation: UNIZOR.COM - Relativity 4 All - Einstein View

Notes to a video lecture on UNIZOR.COM

Length Transformation

This lecture continues the previous ones at UNIZOR.COM - Relativity 4 All - Einstein View. We strongly recommend to familiarize yourselves with these lectures prior to studying the material of this one.

As was described in the previous lectures, there is a difference in time perception of the same process by two observers moving relatively to each other.
The proper time, as observed by an observer of the frame, where the process was actually happening, was shorter than the time perceived by an outside observer in some other inertial frame.

In this lecture we address the perception of space in an analogous situation.

Consider, as before, two inertial reference frames:
α-frame with coordinates {X,Y,Z}, time T and an α-observer positioned at the origin of coordinates {0,0,0} and
β-frame with coordinates {x,y,z}, time t and a β-observer in it, who can measure the length of some rigid rod at rest in this system of coordinates.

At time T=t=0 both reference frames coincide.
Assume that β-frame uniformly moves relatively to α-frame with speed v along α-frame's X-axis, maintaining parallelism of all corresponding axes.

A rigid rod is at rest in β-frame, its proper length measured by β-observer is R.
What would its length be from α-observer's perspective?
Would the result depend on orientation of this rod relatively to a trajectory of β-frame in coordinates of α-frame?

Consider two cases:
(a) a rod is perpendicular to a trajectory positioned along Z- and z-axes and
(b) a rod is positioned along X- and x-axes, that is parallel to a trajectory.

We will use the Lorentz Transformation formulas presented in the previous lectures.

The Lorentz transformation from inertial α-frame coordinates {X,Y,Z,T} to inertial β-frame coordinates {x,y,z,t}, when β-frame is uniformly moving with constant speed v along α-frame's X-axis, as derived in earlier lecture of this course, is:

t =	T − v·X/c² √1−(v/c)²

x =	X − v·T √1−(v/c)²

y = Y
z = Z
where c is the speed of light in empty space - the same constant for all inertial reference frames, as postulated in the Theory of Relativity.

The reverse transformation from coordinates {x,y,z,t} of β-frame to coordinates {X,Y,Z,T} of α-frame that moves relative to β-frame with speed −v along x-axis is:

T =	t + v·x/c² √1−(v/c)²

X =	x + v·t √1−(v/c)²

Y = y
Z = z


The case of a rod perpendicular to a trajectory of β-frame's movement

A rod in β-frame is at permanent position for any time moment t stretching perpendicularly to x-axis along z-axis from point
{x,y,z}={0,0,0}
to point
{x,y,z}={0,0,R}
which makes its proper length in β-frame to be always R.

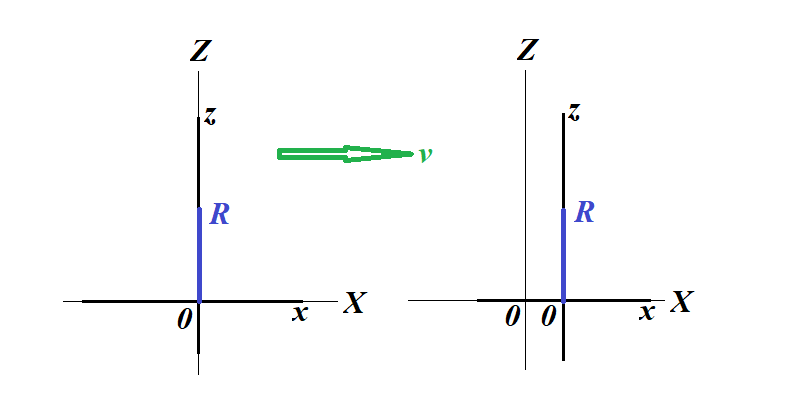
(Click right mouse button to open a bigger picture in a new tab)

Using the transformation of coordinates from β- to α-frame, we obtain the coordinates of this rod in α-frame.

The edge of a rod at β{0,0,0} will have α-coordinates

T0 =

t + v·0/c² √1−(v/c)²

= γ·t

X0 =

0 + v·t √1−(v/c)²

= γ·v·t

Y₀ = y₀ = 0
Z₀ = z₀ = 0
where γ = 1/√1−(v²/c²)

The edge of a rod at β{0,0,R} will have α-coordinates

TR =

t + v·0/c² √1−(v/c)²

= γ·t

XR =

0 + v·t √1−(v/c)²

= γ·v·t

Y_R = y_R = 0
Z_R = z_R = R

From the above expressions for α-coordinates of the edges follows that for any time moment its length in α-frame is the distance from point {X=γ·v·t,Y=0,Z=0} to point {X=γ·v·t,Y=0,Z=R}, which is R.

The perceived length of a rod positioned perpendicularly to a trajectory of its movement is the same as its proper length measured in the reference frame, where this rod is at rest.


The case of a rod parallel to a trajectory of β-frame's movement

Assume that in β-frame the rigid rod of the length R is positioned along x-axis from x=−R to x=0.
As β-frame is moving along X-axis of α-frame, the entire length of a rod is passing in-front of the α-observer positioned at the origin of α-frame.


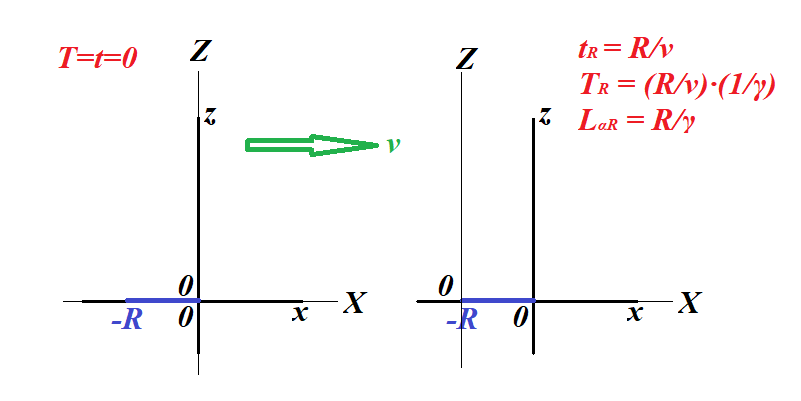
(Click right mouse button to open a bigger picture in a new tab)

Consider two events:
(a) the start of time , when the right end of the rod has coordinates x=0, t=0 in β-frame and X=0, T=0 in α-frame;
(b) the left end of the rod is passing in-front of α-observer, as β-frame moves along X-axis with speed v.

Measuring the time between these two events, as perceived by α-observer, is sufficient to calculate the length of the rod from his viewpoint. All we have to do is to multiply this time by the speed v of β-frame moving relative to α-frame.

The event (a) is, obviously, {X=x=0,Y=y=0,Z=z=0,T=t=0} in both reference frames.
The event (b) occurs when the left end of a rod is passing through point X=0 at some time T_R in α-frame. In β-frame its x-coordinate is still −R. The timing t_R of this event in β-frame is the time β-frame moves along X-axis by the length R of the rod, that is t_R=R/v.

Let's summarize information about event (b):
X_R = 0
T_B is unknown
x = −R
t_R = R/v

The Lorentz Transformation for X-coordinate from β-frame to α-frame is

XR =

−R + v·R/v √1−(v/c)²

= 0

as expected

The Lorentz Transformation for T-coordinate from β-frame to α-frame is

TR =

R/v + v·(−R)/c² √1−(v/c)²

=

=

(R/v)·(1 − v²/c²) √1−(v/c)²

=

= (R/v)·√1−(v/c)² 

Multiplying this timing by the speed of β-frame moving relative to α-frame, we obtain the length L_αR of a rod moving across with speed v, as perceived by α-observer:
L_αR = v·T_R = R·√1−(v/c)² 

Using a traditional symbol
γ = 1/√1−(v²/c²)
(which is greater than 1),
the length, as perceived by α-observer, is
L_αR = R/γ

The length, perceived by an outside observer in some inertial frame, relative to which a rigid rod is moving and is positioned along the trajectory of movement, is shorter than its proper length measured in the frame where this rod is at rest.
.