Wave Equation 1
In order to understand the propagation of oscillation in a medium, in the last lecture we discussed the oscillation of two molecules inside a thin rod.
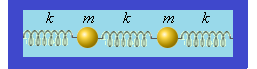
We left our problem with a system of two differential equations describing the displacements x1(t) and x2(t) of these two molecules of mass m each, connected by weightless springs of elasticity k, as functions of time t:
m·x"1(t) + 2k·x1(t) − k·x2(t) = 0
m·x"2(t) − k·x1(t) + 2k·x2(t) = 0
or, using the familiar concept of angular speed ω²=k/m,
x"1(t) + 2ω²·x1(t) − ω²·x2(t) = 0
x"2(t) − ω²·x1(t) + 2ω²·x2(t) = 0
We then suggested a vector form of this system of equations that resembles the equation of harmonic oscillations.
Consider a vector X(t) of displacements
X(t) =
| x1(t) | |
| x2(t) |
and a matrix Ω of coefficients
Ω =
| 2ω² | −ω² |
| −ω² | 2ω² |
Now our system of two differential equations can be represented as one equation with vector argument X(t):
X"(t) + Ω·X(t) = 0
where X"(t) signifies a vector of second derivatives of each component of a vector X(t) and 0 signifies a null-vector.
An operation · (dot) in this equation signifies a multiplication of a matrix Ω by a vector X(t).
Compare it with an equation for harmonic oscillations we discussed in the very first lecture on Waves
x"(t) + (k/m)·x(t) = 0
where later on we assigned ω²=k/m.
Similarity of equations is obvious. Can solution be similar as well?
Multiplication of a vector by a matrix represents a linear transformation of a vector space. This transformation can make a vector longer or shorter, it can turn it to any direction, including an opposite one.
Here is a matrix that stretches any vector by a factor of 5:
| 5 | 0 |
| 0 | 5 |
Indeed, multiplication of this matrix by a vector
| p | |
| q |
| 5·p+0·q | |
| 0·p+5·q |
| 5·p | |
| 5·q |
that is, an original vector stretched in length by a factor of 5.
Here is a matrix that turns any vector by 90°:
| 0 | −1 |
| 1 | 0 |
Indeed, multiplication of this matrix by a vector
| p | |
| q |
| 0·p−1·q | |
| 1·p+0·q |
| −q | |
| p |
that is, an original vector turned counterclockwise by 90°.
There is a big difference between the two examples above.
It's much easier to solve differential equations like our
X"(t) + Ω·X(t) = 0
if matrix Ω is of "stretching" type.
Indeed, if Ω·X(t)=λ·X(t), where λ is constant, our vector equation
X"(t) + λ·X(t) = 0
can be reduced to two independent scalar equations
x1"(t) + λ·x1(t) = 0
x2"(t) + λ·x2(t) = 0
and we know how to solve them.
Unfortunately, the matrix we are dealing with in our problem of two oscillating molecules is not of a "stretching" type.
For example, a unit vector along the X-axis will be turned and unevenly stretched by multiplication by our matrix:
| 2ω² | −ω² |
| −ω² | 2ω² |
| 1 |
| 0 |
| 2ω² |
| −ω² |
But not everything is lost.
Apparently, there are some vectors that are just stretched by this matrix. The plan now is
(a) to find all vectors that are just stretched by our matrix and
(b) try to find partial solutions to our differential equations among only these vectors.
So, we are looking for vectors
V =
| v1 | |
| v2 |
such that
| 2ω² | −ω² |
| −ω² | 2ω² |
| v1 |
| v2 |
| v1 |
| v2 |
or
| 2ω² | −ω² |
| −ω² | 2ω² |
| v1 |
| v2 |
| λ·v1 |
| λ·v2 |
or
| 2ω² | −ω² |
| −ω² | 2ω² |
| v1 |
| v2 |
| λ | 0 |
| 0 | λ |
| v1 |
| v2 |
or
| (2ω²−λ) | −ω² |
| −ω² | (2ω²−λ) |
| v1 |
| v2 |
| 0 |
| 0 |
We cannot be satisfied with a trivial solution of null-vector v1=v1=0 to this system of equations.
To have non-trivial solutions, that is to find non-zero vectors that are stretched by our matrix, the determinant of this matrix must be equal to zero.
So, let's find λ that brings the determinant of this matrix to zero and then non-zero vectors that are stretched by this matrix.
It means, we have to solve an equation for λ:
det
| (2ω²−λ) | −ω² |
| −ω² | (2ω²−λ) |
or
(2ω²−λ)² − (−ω²)² = 0
or (2ω²−λ)² = (ω²)²
or 2ω²−λ = ±ω²
from which we have two solutions:
λ1 = ω² and λ2 = 3ω²
These values are called eigenvalues of matrix Ω.
Potentially, there might be some vectors that our matrix Ω stretches by a factor λ1=ω² without any rotation and there might be some other vectors stretched by Ω by a factor of λ2=3ω².
Let's try to find these vectors because, since they are just stretched (that is, multiplied by a constant) by matrix Ω, we might easily find solutions to our system of differential equations by separating it into two independent equations, one for x1(t) and another for x2(t).
Firstly, let's find such a vector (or vectors) that matrix Ω stretches by λ1=ω².
| 2ω² | −ω² |
| −ω² | 2ω² |
| v1 |
| v2 |
| v1 |
| v2 |
It means that the result of multiplication of a matrix by a vector equals to multiplication of a factor by this vector.
Therefore,
| 2ω²·v1−ω²·v2 |
| −ω²·v1+2ω²·v2 |
| ω²·v1 |
| ω²·v2 |
The above represents two separate scalar equations: 2ω²·v1−ω²·v2 = ω²·v1 and
−ω²·v1+2ω²·v2 = ω²·v2
Both equations produce
v1 = v2
which means that any vector with X-coordinate equaled to Y-coordinate is just stretched by matrix Ω.
Such a vector is called eigenvector.
In this case any vector function of time argument that looks like this
| x(t) |
| x(t) |
is an eigenvector that transformation described by matrix Ω stretches by a factor of λ1=ω².
Indeed,
| 2ω² | −ω² |
| −ω² | 2ω² |
| x(t) |
| x(t) |
=
| 2ω²·x(t)−ω²·x(t) |
| −ω²·x(t)+2ω²·x(t) |
=
| ω²·x(t) |
| ω²·x(t) |
| x(t) |
| x(t) |
Secondly, let's find such a vector (or vectors) that matrix Ω stretches by λ2=3ω².
| 2ω² | −ω² |
| −ω² | 2ω² |
| v1 |
| v2 |
| v1 |
| v2 |
It means that the result of multiplication of a matrix by a vector equals to multiplication of a factor by this vector.
Therefore,
| 2ω²·v1−ω²·v2 |
| −ω²·v1+2ω²·v2 |
| 3ω²·v1 |
| 3ω²·v2 |
The above represents two separate scalar equations: 2ω²·v1−ω²·v2 = 3ω²·v1 and
−ω²·v1+2ω²·v2 = 3ω²·v2
Both equations produce
v1 = −v2
which means that any vector with X-coordinate equaled by absolute value but opposite in sign to Y-coordinate is just stretched by matrix Ω.
All such vectors are also eigenvectors.
In this case any vector function of time argument that looks like this
| y(t) |
| −y(t) |
is an eigenvector that transformation described by matrix Ω stretches by a factor of λ2=3ω².
Indeed,
| 2ω² | −ω² |
| −ω² | 2ω² |
| y(t) |
| −y(t) |
=
| 2ω²·y(t)+ω²·y(t) |
| −ω²·y(t)−2ω²·y(t) |
=
| 3ω²·y(t) |
| −3ω²·y(t) |
| y(t) |
| −y(t) |
Summarizing the above, we have two sets of eigenvectors:
one that is stretched by matrix Ω by a factor of ω² and looks like
{P} =
| x(t) |
| x(t) |
where x(t) is any function of time t,
and another that is stretched by matrix Ω by a factor of 3ω² and looks like
{Q} =
| y(t) |
| −y(t) |
where y(t) is any function of time t.
All of the above analysis leads us to an opinion that we might attempt to look for solutions to a vector form of our differential equation
X"(t) + Ω·X(t) = 0
only among those vectors X(t) that belong to either set {P} of eigenvectors of matrix Ω with eigenvalue ω² or set {Q} of eigenvectors of matrix Ω with eigenvalue 3ω².
Assume, X(t) ∈ {P},
that is X(t) =
| x(t) |
| x(t) |
where x(t) is some unknown function of time t.
Then Ω·X(t) = ω²·X(t) and our equation looks like
X"(t) + ω²·X(t) = 0 or
| x"(t) |
| x"(t) |
| x(t) |
| x(t) |
| 0 |
| 0 |
which is equivalent to one regular differential equation
x"(t) + ω²·x(t) = 0
which is an equation of harmonic oscillations with a general solution
x(t) = D·cos(ωt+φ)
where amplitude D and phase shift φ depend on initial conditions.
Assume, X(t) ∈ {Q},
that is X(t) =
| y(t) |
| −y(t) |
where y(t) is some unknown function of time t.
Then Ω·X(t) = 3ω²·X(t) and our equation looks like
X"(t) + 3ω²·X(t) = 0 or
| y"(t) |
| −y"(t) |
| y(t) |
| −y(t) |
| 0 |
| 0 |
which is equivalent to one regular differential equation
y"(t) + 3ω²·y(t) = 0
which is an equation of harmonic oscillations with a general solution
y(t) = E·cos(√3ωt+ψ)
where amplitude E and phase shift ψ depend on initial conditions.
Now we have two different sets of solutions for our system of two degrees of freedom:
X(t) =
| D·cos(ωt+φ) |
| D·cos(ωt+φ) |
and
Y(t) =
| E·cos(√3ωt+ψ) |
| −E·cos(√3ωt+ψ) |
where D, E, φ and ψ can be any constants and ω²=k/m is a known characteristic of the system..
From the linearity of the differential equations involved follows that any linear combination of solutions from these two sets is also a solutions.
Therefore, a more general expression of solutions is
α·X(t) + β·Y(t)
or (dropping multipliers D and E since they, as well as multipliers α and β, are any numbers)
| α·cos(ωt+φ)+β·cos(√3ωt+ψ) |
| α·cos(ωt+φ)−β·cos(√3ωt+ψ) |
As we see, the motion of our two objects between springs is a superposition of two oscillations with different angular speeds, amplitudes and phase shifts.
Easy check shows that for any α, β, φ and ψ the above vector represents a solution to our vector differential equation
X"(t) + Ω·X(t) = 0
It remains to be proven that this expression encompasses all solutions and, therefore, can be considered as a general solution to differential equations that describe our system of two objects connected with springs, but this proof is a part of a more advanced course of differential equations and is outside of the scope of this course.
Another important part of the analysis is to find all unknown constants (α, β, φ and ψ) based on some initial conditions (displacements and velocities) of oscillating objects. It requires tedious but straight forward calculations and solving algebraic systems of equations, so we leave it anybody with inquisitive mind to do himself.


No comments:
Post a Comment