Speed of Light: UNIZOR.COM - Physics4Teens - Waves - Field Waves

Notes to a video lecture on http://www.unizor.com

Speed of Light

Based on Maxwell equations, we can derive separate differential equations for vectors of electrical (E) and magnetic (B) components of a electromagnetic field and the speed of its propagation in a simple case of vacuum as a medium and no additional sources of electric charges, moving or stationary, present.

As we know, oscillations of electromagnetic field are transverse, vectors E and B are perpendicular to each other and both are perpendicular to the direction of waves propagation.

Assume that the wave propagation is along the Z-axis of some system of coordinates, electric component E is changing along the X-axis and magnetic component B is changing along the Y-axis.


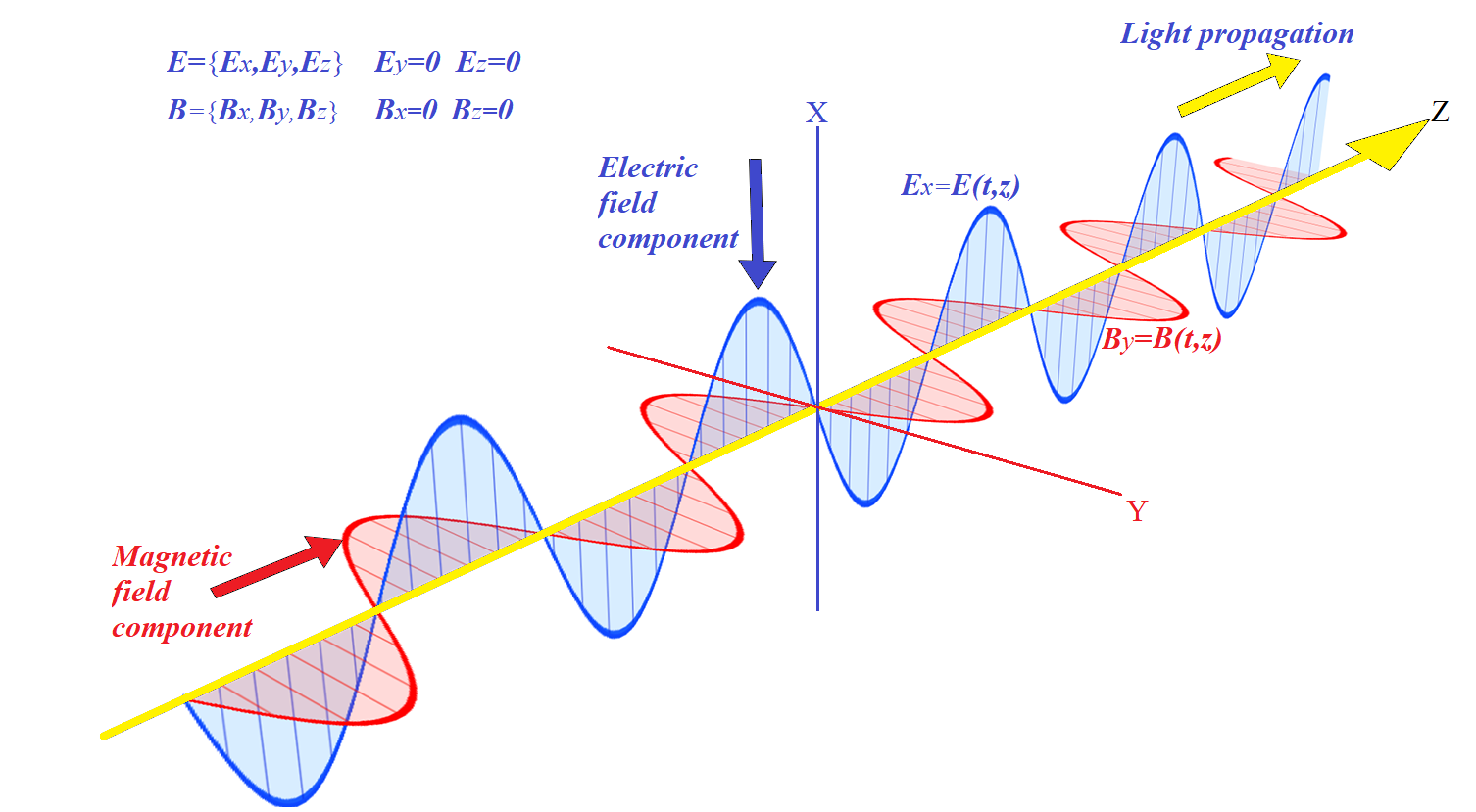
For this type of the electromagnetic field we can state
E = {E_x,E_y,E_z}
with E_y=0 and E_z=0,
while E_x(t,z) being a function of time t and coordinate along the Z-axis z.
Analogously,
B = {B_x,B_y,B_z}
with B_x=0 and B_z=0,
while B_y(t,z) being a function of time t and coordinate along the Z-axis z.

Using the above format of vectors of electrical (E) and magnetic (B) components of the electromagnetic field, we can analyze the form of the Maxwell equations for them.

The third Maxwell equation that relates induced electric field to a changing magnetic field - the Faraday's Law - is

∇⨯E = −∂B/∂t

Recall the definition of a vector (cross) product of a pseudo-vector
∇={∂/∂x,∂/∂y,∂/∂z}
by any vector
V(x,y,z) = {V_x,V_y,V_z}
using unit vectors i, j and k along the coordinate axes:
∇⨯V =
= (∂V_z/∂y − ∂V_y/∂z)·i +
+ (∂V_x/∂z − ∂V_z/∂x)·j +
+ (∂V_y/∂x − ∂V_x/∂y)·k
(you can refresh this in the lecture "Curl in 3D" of this chapter of a course on UNIZOR.COM)

Considering a special characteristics of vector E with only E_x(t,z)≠0,
∇⨯E =
= (∂E_z/∂y − ∂E_y/∂z)·i +
+ (∂E_x/∂z − ∂E_z/∂x)·j +
+ (∂E_y/∂x − ∂E_x/∂y)·k =
= (∂E_x/∂z)·j

Considering a special characteristics of vector B with only B_y(t,z)≠0,
−∂B/∂t = −(∂B_y/∂t)·j

Therefore, the third Maxwell equation in this case of a simple electromagnetic field takes the form
(∂E_x/∂z)·j = −(∂B_y/∂t)·j
Hence,
∂E_x/∂z = −∂B_y/∂t

The fourth Maxwell Equation looks like

∇⨯B = μ·ε·∂E/∂t + μ·σ·E

Here the variables participating in this equation are:
μ is a magnetic permeability of the media,
ε is an electric permittivity of the media,
σ is a material conductivity of the media.

Considering our medium is vacuum, σ=0, and the fourth Maxwell equation looks like
∇⨯B = μ·ε·∂E/∂t
Since only B_y(t,z)≠0,
∇⨯B =
= (∂B_z/∂y − ∂B_y/∂z)·i +
+ (∂B_x/∂z − ∂B_z/∂x)·j +
+ (∂B_y/∂x − ∂B_x/∂y)·k =
= −(∂B_y/∂z)·i

The vector E on the right side of the fourth Maxwell equation has only X-component not equal to zero.
Therefore, this equations looks like
−(∂B_y/∂z)·i = μ·ε·(∂E_x/∂t)·i
Hence,
−∂B_y/∂z = μ·ε·∂E_x/∂t

As a result, the third and fourth Maxwell equations for an electromagnetic field in vacuum with no additional charges take the short form:
∂E_x/∂z = −∂B_y/∂t
−∂B_y/∂z = μ·ε·∂E_x/∂t

To separate electric and magnetic components into separate single variable equations, let's differentiate the first equation by space coordinate z and the second equation - by time t.
∂²E_x/∂z² = −∂²B_y/∂t∂z
−∂²B_y/∂z∂t = μ·ε·∂²E_x/∂t²

From these two equations we can construct one for E_x:
∂²E_x/∂z² = μ·ε·∂²E_x/∂t²

We can drop an index x for an electric component of an electromagnetic field since the other two components are zero.
In vacuum magnetic permeability μ is a known constant, approximately equal to
μ₀=1.25663706212·10⁻⁶
(Henry/meter)
and an electric permittivity ε is also a known constant, approximately equal to
ε₀=8.85418781281·10⁻¹²
(Farad/meter).

In these terms the last equation for the electric component of an electromagnetic field is
∂²E(t,z)/∂z² = μ₀·ε₀·∂²E(t,z)/∂t²
This equation is the general wave equation for the direction of the wave propagation along the Z-axis.

Incidentally, one of the solutions to it, considered in the lecture "Wave Equation 2" of this chapter of the course on UNIZOR.COM is the wave function
E(t,z) = A·sin(ω·(t−z/v))
where ω is the frequency of oscillations and v is the speed of wave propagation.

Indeed, differentiating E(t,z) by z gives
∂E(t,z)/∂z =
= −(ω/v)·A·cos(ω·(t-z/v))
∂²E(t,z)/∂z² =
= −(ω/v)²·A·sin(ω·(t-z/v))

Differentiating E(t,z) by t gives
∂E(t,z)/∂t =
= ω·A·cos(ω·(t-z/v))
∂²E(t,z)/∂t² =
= −ω²·A·sin(ω·(t-z/v))

Therefore,
∂²E(t,z)/∂z² = (1/v²)·∂²E(t,z)/∂t²

If 1/v²=μ₀·ε₀, the wave equation for E(t,z)=A·sin(ω·(t-z/v)) is satisfied.

The last statement is extremely important, since it defines the speed of wave propagation in terms of magnetic permeability and electric permittivity of the medium.

Using the constants of magnetic permeability and electric permittivity of vacuum listed above, the speed of propagation of electromagnetic oscillations for vacuum can be calculated as follows:

1/v² = μ₀·ε₀ =
= 11.1265005604·10⁻¹⁸

v² = 1/(μ₀·ε₀) =
= 0.0898755178·10¹⁸

v = √0.0898755178·10⁹ =
= 299,792,458 m/sec

The speed of light in vacuum is usually denoted as c, so
c = 299,792,458 m/sec

All the above calculations were performed by James Maxwell at the end of 19th century.
At the same time the speed of light was measured relatively precisely, and it was almost exactly the same as the speed of electromagnetic waves propagation calculated above.
That led to a hypothesis that light is the oscillations of electromagnetic field.

The magnetic permeability and electric permittivity of vacuum are not dependent on the frequency of oscillations. That's why the speed of light of any color in vacuum is the same as calculated above.
When the light goes through some material substance (like air or glass), the magnetic permeability and electric permittivity of a medium do depend on the frequency of oscillations and, therefore, the speed of light of different colors varies in material substance, which causes such effects as interference, diffraction or dispersion of light.