Electromagnetic Field Equations 4
This lecture is about the Fourth Maxwell equation, expressing in differential form the Ampere-Maxwell's Law - relationship between a changing in time electric field and produced by this process changing in time magnetic field.
But, before diving into mathematical concepts of the Fourth Maxwell's Equation, some general discussion is appropriate.
We mentioned previously that a changing magnetic field induces a changing electric field (Faraday's Law), which was expressed in the Third Maxwell's Equation.
Ampere's Law describes the magnetic field generated by an electric current, actual movement of electric charges.
Constant electric current generates constant magnetic field, variable current generates variable magnetic field.
But constant magnetic field will not generate an electric field. For propagation of electromagnetic field the initial electric current must be variable to produce a variable electric field, which will generate a variable magnetic field, which, in turn, generate a new variable electric field etc.
The addition of an impact of the variable electric field onto a generated magnetic field was introduced by James Maxwell in his Fourth Equation.
If we consider only transformations between variable electric and magnetic fields, it looks like the electric and magnetic components of an electromagnetic field are symmetrical, and the equation, expressing how variable electric field generates variable magnetic field (the Fourth Maxwell equation), should be symmetrical to the Third Maxwell equation describing how changing magnetic field generates a changing electric field.
But it's not exactly so.
We do need some initial source of energy, a variable electric current, to start this chain of transformations between electric and magnetic fields.
While changing electric field generates changing magnetic field and thus propagates through oscillations of a combined electromagnetic field, the first originating changing electric field in this chain (before the first changing magnetic field generated) should appear from somewhere, and that "somewhere" is the flow of electrons - a movement of material objects in our everyday's understanding of the word "material", which is different from "field".
The generation of a magnetic field must include two sources: electric current component capable of generation of magnetic field (constant or changing) and a variable electric field component generating variable magnetic field.
We will address both components separately and then present their sum as the combined source that generates a variable magnetic field.
The Fourth Maxwell Equation encompasses both effect on a generated magnetic field by electric current (Ampere's Law) and by a variable electric field (Maxwell's addition).
We start with magnetic field generated by an electric current.
The existence of a magnetic field around an electric current was introduced in the "Electromagnetism" part of this course, in the topic "Magnetism of Electric Current", which we strongly recommend to review.
Recall the familiar experiment of iron shavings positioned around a straight line electric wire with a current running through the wire that results in creation of visible magnetic field lines in circular formation around a wire.
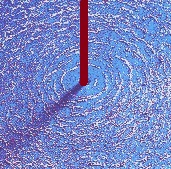
There is no other source of magnetism that can cause this formation, so we must conclude that an electric current creates a magnetic field that moves iron shavings into circular positions.
In the same topic "Magnetism of Electric Current" mentioned above we suggested the logical basis for a formula for an intensity of the magnetic field around an infinite straight line electric current as dependent on the amperage of the electric current and a distance from a wire carrying this current:
B = μI/(2πR)
where
B is an intensity of the magnetic field,
I is an electric current,
R is a distance of a point of measurement of the magnetic field intensity from the wire with a current,
μ is a magnetic permeability of the media.
Notice that from this formula follows
μ·I = B·(2πR)
That is, an electric current I going through a circular loop times the magnetic permeability of the media (μ0 for vacuum) equals to a circulation of a magnetic field generated by this current along its loop.
This description represents a particular case of a more general Ampere's Circuital Law that states that for any closed loop L around an electric current I the circulation of the magnetic field produced by this current equals to the electric current enclosed in the loop times a magnetic permeability constant:
μ·I = ∫[L]B·dL
Indeed, if the path L is a circle of radius R in the plane perpendicular to a straight line conductor with an electric current I flowing through it, vectors B and dL are collinear with the length of B constant. Therefore, the integral above would be equal to a product of a constant magnitude of a magnetic field intensity by the length of a circumference of radius R:
μ·I = B·(2πR).
To transform this equation into point-based differential one, let's perform two operations:
(1) we will squeeze the loop around point {x,y} on the plane perpendicular to a direction of an electric current and
(2) we will divide both sides of an equation above, the electric current on the left and the circulation on the right, by an area of the loop of a magnetic field line around point {x,y}
As a result of these steps, as presented in the lecture about circulation, the ratio of a circulation to an area of the loop will tend to a curl of a magnetic field at point {x,y}.
On the other hand, division of the electric current by the same area produces average density of electricity flowing through the loop, which in limit, when the loop is squeezed to a point, will produce the density of electricity at that point {x,y}.
This leads us to an equation for point {x,y} in the magnetic field
curl B(x,y) = μ·J(x,y)
where J(x,y) is a density of electric current at point {x,y}.
As we know,
curl B(x,y) = ∇⨯B(x,y), which allows to right the equation above as
∇⨯B(x,y) = μ·J(x,y)
We might want to engage the electric field intensity E instead of current density J into our equation. To accomplish this, we can make one more variation of the above equation based on the empirical Ohm's Law.
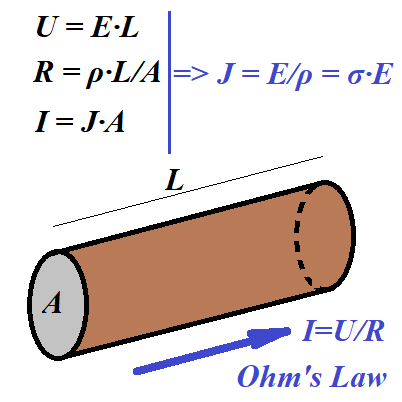
Recall that Ohm's Law states
U = I·R
where U is difference in potential,
I - electric current,
R - resistance.
Resistance R is proportional to length of a conductor L and inversely proportional to the area A of its cross-section:
R = ρ·L/A
where ρ is resistivity of the material of a conductor, that is the resistance of the unit of area per unit of length of a conductor.
The conductivity σ is an inverse of ρ.
Therefore,
R = L/(σ·A)
where A is the area of a conductor,
L is the length of a conductor.
Therefore, the Ohm's Law can be written as
U = I·L/(σ·A)
or
U/L = (I/A)/σ
The expression I/A is the electric current density J.
Since U is the difference in potential, it's the work to transfer a unit of electric charge from one end of a conductor to another.
Since work is the force times distance, and the force acting on a unit charge in the electric field is the field's intensity E,
E = J/σ
σ·E = J
Now we can replace the current density J with a product of electric field intensity E and material conductivity σ: J=σ·E, which transforms the equation above into
∇⨯B(x,y) = μ·σ·E(x,y)
This expression for a curl of a magnetic field produced by electric current constitutes the half of the Fourth Maxwell's Equation.
The second half depends on changing electric field generating a changing magnetic field.
First of all, let's support by an experiment that a changing electric field generates a changing magnetic field.
Consider a capacitor.
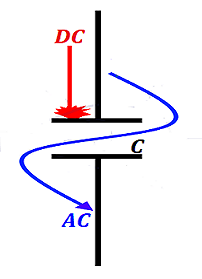
The alternating current (AC) in the circuit goes through a capacitor connected to some generator of AC power.
The amount of electricity Q(t) accumulated on each plate of a capacitor is proportional to a variable voltage U(t) applied to its plates, and capacity of a capacitor C (see lecture "Electric Fields" - "Capacitors" in this course) is the constant proportionality factor that depends on a type of a capacitor C = Q(t)/U(t)
Q(t) = C·U(t)
Knowing the amount of electricity Q(t) accumulated in a capacitor as a function of time t, we can determine the electric current I(t) in a circuit, which is a rate of change (that is, derivative by time) of the amount of electricity
I(t) = dQ(t)/dt = C·dU(t)/dt
This I(t) is not as "material" as a current in a wire, no electrons are exchanged directly between the plates, but, nevertheless, it's real and, as the "material" current in a wire, it generates a magnetic field around it.
There is a special name for this electric current - displacement current, and we will use the symbol ID(t) for it to differentiate it from the electric current caused by actual movement of charges.
Now we will follow the logic presented above for wire-based electric current generating a magnetic field to calculate the effect of the displacement current, which is a product of the changing electric field without actual movement of the charges.
First of all, we will evaluate the displacement current as follows
ID(t) = C·dU(t)/dt
Capacity C, as described in the lecture "Electric Fields" - "Capacitors" in this course is
C = A·ε/d
where A is the area of a plate of a capacitor (that is, the area, through which displacement current is flowing),
ε is the permittivity of the media between the plates,
d is the distance between the plates.
Therefore,
ID(t) = (A·ε/d)·dU(t)/dt =
= A·ε·d[U(t)/d]/dt
If E(t) is the intensity of the electric field between the plates with voltage U(t) between them,
E(t) = U(t)/d
From this follows
ID(t) = A·ε·dE(t)/dt
In terms of density of the displacement current
JD(t)=ID(t)/A
this looks like
JD(t) = ε·dE(t)/dt
Let's consider now any changing in time electric field of intensity E(t,x,y) in two-dimensional XY-coordinate space.
We can imagine a tiny capacitor with plates parallel to XY-plane at point {x,y}, for which the equation above, which is independent on the size of the plates and the distance between them, represents a displacement current density.
Now the expression above for a displacement current density can be stated for any point {x,y} as
JD(t,x,y) = ε·∂E(t,x,y)/∂t
Using the equation above for a curl of a magnetic field intensity as a function of electric current density
curl B(x,y) = μ·J(x,y),
we evaluate another part of a total magnetic field intensity, which depends on variability of the electric field and displacement current density:
curl B(x,y) = μ·JD(t,x,y) =
= μ·ε·∂E(t,x,y)/∂t
Combining both components of the magnetic field intensity, one that depends on the moving charges and another that depends on variability of the electric field, we obtain the Fourth Maxwell Equation
curl B(t,x,y) =
= μ·ε·∂E(t,x,y)/∂t + μ·σ·E(t,x,y)
Using our favorite symbol ∇ for curl, the Fourth Maxwell Equation looks like
∇⨯B(t,x,y) =
=μ·ε·∂E(t,x,y)/∂t + μ·σ·E(t,x,y)


No comments:
Post a Comment