Geometry+ 07: UNIZOR.COM - Math+ & Problems - Geometry

Notes to a video lecture on http://www.unizor.com

Geometry+ 07

Problem A

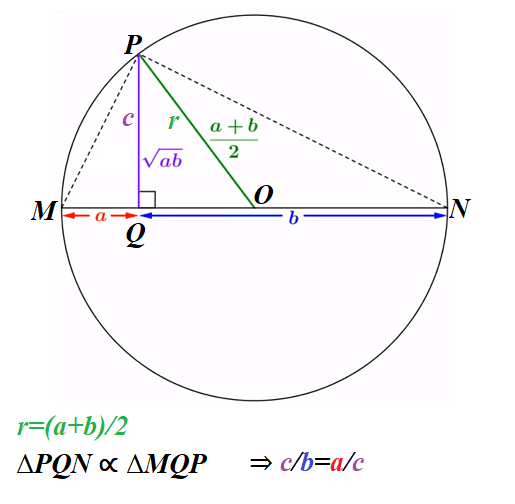
Given any circle with a center at point O, its diameter MN and any point P on this circle not coinciding with the ends M, N of a given diameter.
Let point Q be a projection of point P on a diameter MN.
This point Q divides diameter MN into two parts:
MQ = a and QN= b

Prove that
(1) Radius of a OP circle is an arithmetic average of a and b.
(2) Projection segment PQ is a geometric average of a and b.
(3) Based on these proofs, conclude that geometric average of two non-negative real numbers is less or equal to their arithmetic average.

Proof:
(1) Diameter MN of this circle
is 2r=a+b.
Therefore,
r = ½(a+b) - arithmetic average of a and b.
(2) ΔMPN ∝ ΔPQN ∝ ΔMQP
as right triangles with congruent angles.
⇒ c/b=a/c
⇒ c² = a·b
⇒ c = √a·b - geometric average of a and b
(3) Cathetus c is smaller than hypotenuse r in ΔPQO.
Therefore,
√a·b = c ≤ a = ½(a+b)
End of the proof.


Problem B

Prove the following theorem:
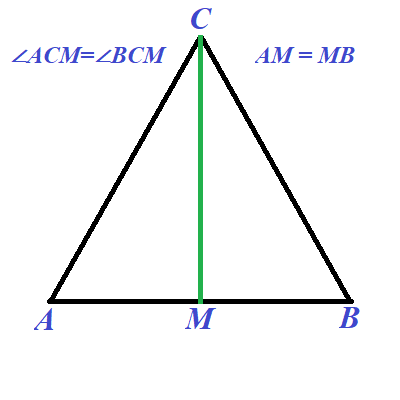
If a median and an angle bisector in a triangle coincide, then this triangle is isosceles.

Important
Triangle ΔACM has two sides congruent to corresponding two sides of triangle ΔBCM because they share side CM and AM=BM since CM is a median.
They also have congruent angles ∠ACM=∠BCM since CM is an angle bisector.
But we cannot use a theorem about triangles ΔACM and ΔBCM being congruent by two sides and an angle because the angle is not between two congruent sides.
See the lecture Geometry+ 01 of this course, Problem A as an illustration of a case when two sides of one triangle are equal to two sides of another one and all angles of the first triangle are equal to all angles of the second, yet these triangles are not congruent.

Hint
Extend segment CM beyond point M to point N such that CM=MN.

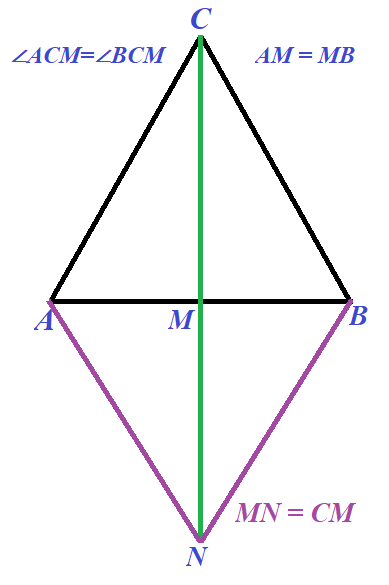
Prove that ΔAMN = ΔBCM.
Then prove that ΔACM is isosceles.


Problem C

Given a convex polygon with N vertices.
Draw all the possible diagonals in it.
(1) Assuming that no three diagonals intersect at the same point, how many points of intersection between diagonals will exist?
(2) What is the sum of all angles inside this polygon formed by all its intersecting diagonals and sides?

Hint C

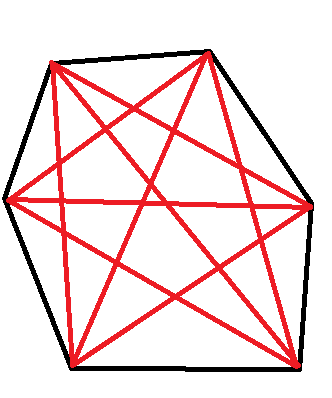
For N=6 the number of intersections is 15.
Consider quadrilaterals formed by any four vertices.

Answer C
The number of intersections between the diagonals is
n = (N!)/ [(4!)·(N−4)!]
The total sum of all angles inside a polygon is equal to
(2n+N−2)·π