Trigonometry+ 04: UNIZOR.COM - "Math+ & Problems" - "Trigonometry"

Notes to a video lecture on http://www.unizor.com

Trigonometry+ 04

Problem A

Find the sums
Σ_{k∈[0,n−1]}sin²(x+k·π/n)

Solution A

First, convert sin²(...) into cos(...) by using the identity
cos(2φ) = cos²(φ) − sin²(φ) =
= 1 − 2sin²(φ)
from which follows
sin²(φ) = ½(1 − cos(2φ))

Now our sum looks like this
Σ_{k∈[0,n−1]}
½(1−cos(2x+2k·π/n)) =
= n/2 −
− ½Σ_{k∈[0,n−1]}cos(2x+2k·π/n)

To calculate Σ_{k∈[0,n−1]} above, let's use the result of the previous lecture Trigonometry 03 that proved the following
Σ_k∈[0,n]cos(x+k·y) =
= [sin(x+(2n+1)·y/2) −
− sin(x−y/2)] /
/ [2·sin(y/2)]

Since in our sum we have n terms instead of n+1, as in the formula above, to use this formula in our case we have to use n−1 instead of n, which will result in
Σ_{k∈[0,n−1]}cos(x+k·y) =
= [sin(x+(2n−1)·y/2) −
− sin(x−y/2)] /
/ [2·sin(y/2)]

To use this formula in our case, we have to substitute 2x instead of x and 2π/n instead of y.

In this case the numerator in the formula above would be
sin(x+(2n−1)·π/n) − sin(x−π/n)

The first term in the above expression for the numerator equals to
sin(x+(2n−1)·π/n) =
= sin(x+2n·π/n−π/n) =
= sin(x−π/n+2π) = sin(x−π/n)
(since the value 2π is a period of function sin)
which makes the first term exactly the same as the second term and they will cancel each other resulting in zero in the numerator.

That leaves the sum in our case to be equal to
Σ_{k∈[0,n−1]}
½(1−cos(2x+2k·π/n)) = n/2

Answer A
Σ_{k∈[0,n−1]}sin²(x+k·π/n) = n/2


Problem B

Given a regular n-sided polygon with vertices A₁, A₂,...,A_n inscribed into a circle of radius R with a center at point O and any point P on this circle.


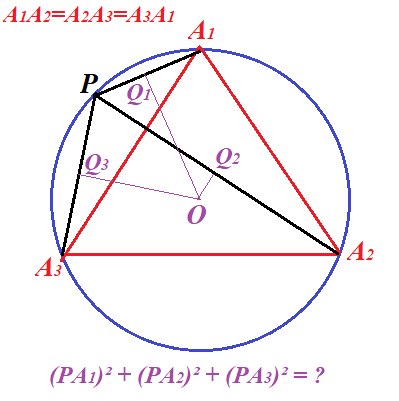
Calculate the sum of squares of distances from point P to all vertices A_k
S = Σ_k∈[1,n](PA_k)².

Hint:
Let ∠POA_k = φ_k.
Then φ_k = φ₁ + (k−1)·2π/n.
Use the results of Problem A.

Answer
S = 2nR²