Notes to a video lecture on http://www.unizor.com
Torque
So far we were mostly considering translational motion of point-objects - a motion along a straight line with or without external forces
acting upon this object. We have specified three Newton's Laws of this
motion and derived a lot of interesting facts based on these laws.
Let's recall these laws.
The Newton's First Law is the familiar Law of Inertia that states that
an object at rest stays at rest and an object in uniform motion stays in
this uniform motion, unless acted upon by unbalanced forces.
The Newton's Second Law brings quantitative relationship to vector of force (F), mass (m) and vector of acceleration (a):
F = m·a
The Newton's Third Law states that for every action there is an equal in magnitude and oppositely directed reaction.
Rotational motion obeys the rules in many respects analogous to the laws of translational motion, except we have to change linear movement to rotation, which, in essence, is an angular movement with constant radius.
Consider a point-object of some mass m connected by a weightless rigid rod of the length r to an axis, around which this object can rotate within a plane of rotation that is perpendicular to an axis of rotation.
The picture below illustrates such a movement and also indicates the position of angular velocity ω
of a rotation, which was explained earlier, when we studied the
Kinematics of rotation (we assume your familiarity with this topic and
strongly recommend to refresh it before proceeding any further).
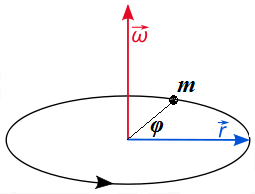
Let's discuss the similarities and differences between translational movement along a straight line and rotation around an axis within a plane of rotation perpendicular to this axis.
Obviously, the time concept remains the same in both types of motion.
There is a clear rotational analogy to the Newton's First Law.
An object at rest stays at rest and an object in uniform rotation
stays in this uniform rotation, unless acted upon by unbalanced forces.
The Newton's Third Law is not really specific for a form of motion, so there is no need to address it separately at this moment.
The Newton's Second Law requires certain modification to be applied to rotation.
Let's address the main concept of Dynamics - the force - in connection to rotation.
The very important characteristic of force in Dynamics of translational movement along a straight line is that it can be measured by its effect on objects of certain inertial mass. Thus, a measure of force that gives linear acceleration of
The same force applied to different objects of the same inertial mass will cause the same linear acceleration etc.
The situation with rotational movement is not the same.
Consider a simple experiment of opening a door. If you apply a force to
open a door at its edge opposite to hinges, where the handle is usually
located, it opens relatively faster than if you apply exactly the same
force in the middle of a door. The closer a point of application of the
same force to hinges - the slower a door opens, if the force applied to
it is the same. In an extreme case, when we apply the pressure where the
hinges are, a door will not open at all.
As a continuation of this experiment, if we want to achieve the same
speed of the opening of a door by applying the force at different
distances from the hinges, we need more efforts for a point of force
application located closer to the hinges.
We can measure the force, the distance from the hinges of a point of application of this force and an angular acceleration of the door and experimentally come up with the fact that for the fixed force the angular acceleration of the door is proportional to the distance of a point of application of the force from the hinges.
Moreover, leaving the point of application of the force the same and changing the force, we can determine that angular acceleration is proportional to the force.
What it means is that in a case of rotation a force by itself does not
determine the final motion of a rotating object. It's a product of a
force F and radius to a point this force is applied r, that determines the final effect. This product
| Translation | Rotation |
| Force F | Torque τ = F·r |
Returning to a picture above, we can apply some force F to
any point on the rigid rod, connecting our point-object to an axis of
rotation, directing this force perpendicularly to the rod, and observe
that the resulting angular acceleration of the object is proportional to
both the force F and the distance r from the axis to a point of application of this force, thus proportional to torque
Now we have concluded that an angular acceleration α of rotational motion is proportional to a torque τ. This is analogous to linear acceleration a of translational motion being proportional to a force F. The coefficient of proportionality for translational motion is inertial mass m of an object (this is the Newton's Second Law F=m·a).
The obvious question is, what is the coefficient of proportionality between angular acceleration and torque?
Answer to this question will result in rotational equivalent of the Newton's Second Law.
We have experimentally established that equal torques produce equal angular accelerations.
Consider a rotation illustrated on the picture above. Assume that the point of application of force F is exactly at the point-object of mass m rotating around an axis at a distance r
from it on a rigid weightless rod. Assume further that our force acts
within a plane of rotation and directed perpendicularly to the rod.
During an infinitesimal time interval dt the motion of an object can be considered as linear and, therefore, the Newton's Second Law can be applied, giving F=m·a.
Now we can express it in terms of angular acceleration and torque as follows:
a = r·α
τ = F·r
Hence,
F·r = (m·a)·r = m·r²·α
Finally,
τ = (m·r²)·α
One more logical step is needed. We started from a force applied on an object itself at a distance r
from an axis. But we have experimentally established that equal torques
produce equal actions. It means that some other force applied to some
other point will produce the same effect, causing the same angular
acceleration, as long as the torque is the same.
So, the equality τ=(m·r²)·α is universal, regardless of point of application of force since it depends not on force, but on torque.
The above equality represents the rotational analogue of the Newton's Second Law.
Some generalization can be applied to the above.
What if the force, acting within a plane of rotation, is not perpendicular to a rod?
Obvious solution is to replace vector F
with its projection onto a line on the rotation plane that is
perpendicular to a radius and to multiply the product of two scalars F·r by a sine of an angle between corresponding vectors, effectively using a vector product F⨯r.
So, more general definition of a torque is a vector (or, more precisely, pseudo-vector)
τ = F⨯r
whose direction, like a direction of an angular acceleration, is along an axis of rotation.
So, for rotational movement the vectors of torque τ and angular acceleration α are collinear, similarly to collinearity of vectors of force F and linear acceleration a for translational movement.
We'd like to note that for purposes of simplicity in this course we will
rarely deal with forces not perpendicular to a radius of rotation.
IMPORTANT TERMINOLOGY POINTS
1. The torque τ is often called the moment of force.
2. Recall the expression tying together a torque τ and an angular acceleration α
τ = (m·r²)·α
The expression m·r² is called moment of inertia, is symbolized by letter I, which allows to specify the formula above as
τ = I·α
where I=m·r² is a moment of inertia, playing the same role in this equation as inertial mass m in the Newton's Second Law and representing resistance to a rotational force.
| Translation | Rotation |
| Force F | Torque τ = F·r |
| Acceleration a | Angular Acc. α = a/r |
| Inertial Mass m | Moment of Inertia I = m·r² |
| Newton's Second Law F=m· | Rotational Equivalent τ = I·α |


No comments:
Post a Comment