Energy of Waves
Any waves can do work. Water waves lift the ships up and down. Sound waves moves eardrums, so we hear sound. Light waves in a form of sun rays heat objects.
All these examples indicate that all waves carry some energy with them.
Light is the oscillations of electromagnetic field. But, prior to investigating these particular types of oscillations, let's spend some time discussing oscillations in general, starting from mechanical oscillation of a point mass on an ideal spring.
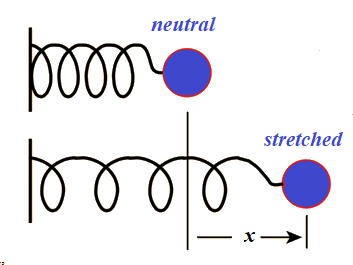
The Hooke's Law for an ideal spring states that the force of a spring F is proportional to an amount of stretch or squeeze x and always directed towards a neutral position
F(x) = −k·x
where the factor k is a characteristic of a spring.
Assume, we are stretching a spring from its initial position by a length A with future plans to let it go from that point to freely oscillate.
Stretching it from length x to length x+dx against the resistance of a spring requires work
dW = −F(x)·dx = k·x·dx
The total amount of work needed to stretch a spring by length A can be calculated by integrating this from 0 to A
W(A) = ∫[0,A]k·x·dx = ½k·A²
If we have stretched a spring by length A doing ½k·A² amount of work and hold the spring in that position, obviously, a spring has a potential energy equal to that amount of work. So, potential energy depends only on how much we stretch or squeeze a spring and its physical characteristics that determine the coefficient of elasticity k.
If we let it go, it will start contracting, increasing the kinetic energy of a point mass on its end and decreasing its potential energy.
Let's calculate this kinetic energy, based on the spring force and the mass m of an object attached to its end.
According to the Newton's Second Law, if x(t) is a distance of the end of a spring with a mass m attached to it from its neutral position, as a function of time t,
F = m·d²x/dt²
On the other hand, according to the Hooke's law,
F = −k·x(t),
where a coefficient of elasticity of a spring k, as we mentioned, is a constant that characterizes the spring.
Therefore, we have a differential equation for x(t)
−k·x(t) = m·d²x/dt²
It's a second order differential equation. To exactly define a solution we need two initial conditions, which are:
x(0) = A since before letting a spring to oscillate we have stretched it at time t=0 by a distance A from a neutral position;
x'(t) = 0 because at time t=0 we just let it go without any initial speed.
Without getting into details of how to solve it (that was covered in details in the course "Math 4 Teens" on this Web site UNIZOR.COM for those interested), we offer a solution
x(t) = A·cos(ω·t),
where ω=√k/m.
Now we can determine the kinetic energy of a moving object on the end of a spring:
K(t) = ½m·v²(t)
Speed v(t) is the first derivative of a distance x(t), that is
v(t) = dx(t)/dt = −A·ω·sin(ω·t)
Therefore, kinetic energy is
K(t) = ½m·A²ω²·sin²(ω·t) =
= ½A²k·sin²(ω·t)
Potential energy at the same moment depends only on the distance x(t) of the spring's end from the neutral position. Its value for distance A we have determined as ½k·A². Analogously, for distance x(t) its value is
P(t) = ½k·x²(t) =
= ½k·A²·cos²(ω·t)
The total energy E at any moment of time t is a sum of potential energy of a spring and kinetic energy of a mass attached to its end:
E = K(t) + P(t) =
= ½A²k·sin²(ω·t) +
+ ½A²k·cos²(ω·t) = ½A²k
The last value equals to the work W(A) we have spent initially to stretch a spring by a distance A from its neutral position and is a constant independent of time t.
So, not surprisingly, we have come to a confirmation of the Energy Conservation Law for oscillating spring.
Initially, all the energy of a system spring + point mass at its end is a potential energy of a spring. As we release a spring to free oscillations, as it returns to a neutral position, its potential energy P(t)=½A²k·cos²(ω·t) diminishes, reaching zero when the end of a spring returns to a neutral position, while the kinetic energy of a point mass K(t)=½A²k·sin²(ω·t) increases, reaching its maximum at the same point.
After passing the neutral point the process reverses, potential energy grows to its maximum, when the distance of the end of a squeezed spring is at distance A from a neutral position, while the kinetic energy diminishes to zero at that point.
The oscillations continue along the same pattern to infinity.
Note that the oscillations propagate along the axis of a spring. An object at the and of a spring moves along the same axis as well.
That's why these oscillations are called longitudinal.
The next lecture will be dedicated to oscillations of a rope. The movements of a horizontally stretched rope with waves forced on it by periodic movement of its edge up and down are more complicated than those of an ideal spring. Not only each small part of a rope moves up and down similarly to oscillations of a spring, but there is a wave front moving along a rope. This complicated movement is close to oscillations of electromagnetic field.


No comments:
Post a Comment