Rope & Springs
A wave on a rope, initiated by some forceful oscillations of its end, propagates along a rope, thereby transferring the initial boost of energy to distant locations.
Our task is to determine how much energy is carried by a single wave on a rope - a part of a rope from crest to crest or from trough to trough, the length of which is the wavelength λ of rope's oscillations.
Let's define the X-axis along the direction the rope is stretched (we will call it "horizontal"). The forced oscillations of the rope's end will be along the Y-axis ("vertical") that is perpendicular to X-axis.
Previous lecture was dedicated to analysis of the vertical displacement of each piece of a rope as a function y(x,t) of its distance from the endpoint of a rope x and time t, if the rope's end (x=0) is forced to perform harmonic oscillations of the form y(0,t)=A·sin(ω·t). Here A is an amplitude and ω is the angular speed of oscillations.
The result of this analysis was a wave equation that function y(x,t) must satisfy
(μ/T)·∂²y(x,t)/∂²t = ∂²y(x,t)/∂x²
where
μ is linear mass density of a rope (mass per unit of length), assumed to be uniform;
T is rope's tension;
The solution to this equation with initial condition y(0,t)=A·sin(ω·t) was suggested in the previous lecture as
y(x,t) = A·sin(ω·t−k·x)
where A and ω are defined above and constant k must satisfy the condition
k²/ω² = μ/T
For the purposes that will be clear later we would like to start time (t=0) not when a rope's end is at horizontal level (y=0), but when it's lifted up to a full extent of an amplitude A, that is our initial condition will be y(0,t)=A·cos(ω·t).
From the energy perspective this modification does not change anything because we will be dealing with an energy of a single wave from crest to crest or from trough to trough, which is the same, whether the wave is based on function sin() or cos(), as long as amplitude A and angular speed ω are the same.
Then the solution to a wave equation that satisfies our initial condition can be
y(x,t) = A·cos(ω·t−k·x)
The approach we will take is based on the analogy between sinusoidal (harmonic) movement up and down of each infinitesimal piece of a horizontally stretched rope and harmonic oscillation of the point-mass object on a vertically oriented spring.
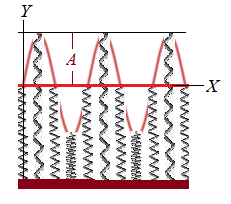
Assume that each infinitesimal piece of a rope of the length dx and mass μ·dx can be considered as an independent point-mass on some tiny spring, and all these pieces are oscillating in a manner resembling and totally identical by all parameters to movements of these pieces as when they are parts of a rope - the same oscillations in terms of the amplitude and angular speed as well as timing of these oscillations.
If all the motion parameters of each infinitesimal piece of a rope are the same as those of the corresponding point-mass on a spring, the forces must be the same and, consequently, the kinetic and potential energy are the same.
Since we have addressed the energy of a point-mass on a spring in earlier lectures (see "Energy of Waves"), we will use these results to calculate the energy of each infinitesimal piece of a rope, which, in turn, will allow to determine the energy of a single wave by integrating all the individual infinitesimal quantities of energy.
Let's start with the equation describing the vertical movement of a rope at each its point that satisfies the wave equation and the initial condition we have set
y(x,t) = A·cos(ω·t−k·x)
where
A is an amplitude (known, defined by external force that causes oscillations);
ω is an angular speed (known, defined by external force that causes oscillations),
t is time;
x is a known distance of the point of a rope that we analyze from its end that is forced to harmonically oscillate to make waves along the rope;
k is a coefficient that depends on the properties of a rope (linear mass density and tension) as well as the angular speed of oscillations ω and defined by an equation
k²/ω²=μ/T,
that is a known quantity equaled to ω·√μ/T .
The physical meaning of the coefficient k was discussed in the previous lecture, that derived the formula for a speed of propagation of a wave v:
v = ω/k.
At the same time speed of wave propagation v is the wavelength λ divided by period or, since a period is an inverse of frequency f, v=λ·f=λ·ω/(2π),
from which we derive
k = ω/v = 2π/λ
v = ω·λ/(2π)
From the formula for a speed of waves propagation we can derived another form of the solution to a wave equation
y(x,t) = A·cos(ω·t−k·x) =
= A·cos[ω·(t−k·x/ω)] =
= A·cos[ω·(t−x/v)]
The expression x/v is, obviously, the time it takes for the wave to go from the place of its origination (x=0) to any fixed distance x, that is a time delay of oscillations at point x from oscillations at point x=0.
So, oscillations at any point x of a rope are the same in terms of amplitude A and angular speed ω, as at point x=0, just with some delay x/v, which depends on a known distance from the wave origination x and a known speed of wave propagation v.
Obviously, we can make a tiny spring with infinitesimal piece of a rope attached to it that has the same oscillations as that same piece being a part of a rope.
We do know the mass attached to a spring, that is the mass of an infinitesimal piece of a rope dx, it's dm=μ·dx.
We can arrange the oscillations of a spring to have the same amplitude A as each piece of a rope. All we need to do is to initially stretch a spring by A.
We also need to have a spring with proper elasticity ke to make sure the angular speed of oscillations of a spring is equal to angular speed of oscillations of each piece of a rope ω.
NOTE: we use here symbol ke for elasticity to differentiate it from k used in the solution for a wave equation y(x,t)=A·cos(ω·t−k·x).
We know from previous lectures that for a spring the angular speed of oscillations relates to elasticity ke and mass of an object attached to it m as
ω² = ke/m.
In our case of a tiny spring with mass attached to it equaled to dm=μ·dx we can determine the elasticity from this mass and required angular speed ω:
ke = ω²·μ·dx
and choose a spring with this exact elasticity.
So, taking a tiny spring with the above elasticity and stretching it by initial length A from its neutral state will produce the oscillations with an amplitude A and angular frequency ω.
What remains to be done is to arrange a proper timing, so the oscillations of the tops of springs will go in waves similarly to waves on a rope.
To accomplish that, after initial stretching of all springs by an amplitude A, we have to release these springs with a time delay required for a wave to reach any particular point on a rope. For a distance x of a particular spring from the beginning, knowing the speed of wave propagation on a rope v, we will just release the spring after x/v time interval from the moment of releasing the first spring.
The movement of the point-mass attached to a spring with described above parameters and delayed start is described by an equation
y(x,t) = A·cos[ω·(t−x/v)]
In the above equation for a spring at distance x amplitude A and angular speed of oscillations ω are defined by initial condition of oscillating the rope
y(0,t) = A·cos(ω·t)
The speed of propagation v is a derived parameter and, therefore, is known as well.
As specified above,
v = √T/μ ,
where μ is a linear mass density of a rope and T is a rope's tension - all known parameters.
Since our task is to determine an energy of a wavelength λ of a rope, we have to integrate the energy of all springs by x on a segment of the length λ. Periodicity of oscillations assures that all such segments will have the same energy.
Let's summarize the characteristics of tiny springs that should oscillate exactly like a rope.
1. Mass attached to each tiny spring equals to dm=μ·dx.
2. Each tiny spring is located at a distance x from the beginning.
3. All springs have the same elasticity ke=ω²·μ·dx
4. All springs are initially stretched by A, so their amplitude will be the same as that of the rope.
5. All springs will have the same angular speed ω, the same as waves on a rope.
6. After initial stretch the springs will be let go, but not simultaneously; the time delay of a spring at distance x from the beginning will correspond to time needed for a wave on a rope to reach that distance, that is the time delay will be equal to x/v=2π·x/(λ·ω).
7. As a result, a spring at distance x from the beginning oscillates according to a formula y(x,t)=A·cos[ω·(t−2π·x/(λ·ω))].
The above characteristics of the springs, that we have used to model the propagating waves on a rope, are sufficient to analyze the distribution of energy, using what we have already analyzed about springs.
Next lecture will use all this to find potential and kinetic energy of a single wave on an oscillating rope.


No comments:
Post a Comment