Interference of Light: UNIZOR.COM - Physics4Teens - Waves - Phenomena of...

Notes to a video lecture on http://www.unizor.com

Interference of Light

Before examining the phenomena of interference of light (recall that light is transverse waves in the electromagnetic field), to clarify this issue, let's start with a simple experiment of transverse waves on a surface of water, produced by two independent sources of oscillation positioned close to each other.

Each source of oscillations, by itself, produces concentric waves on the surface of water with a circular wave front, gradually expanding its radius with some speed of propagation.

Let's introduce a Cartesian system of coordinates with XY-plane coinciding with the water surface in its neutral (without waves) state, Y-axis going along a line connecting two sources of oscillation that have XY-coordinates (0,s) and (0,−s), and Z-axis going perpendicularly to the water surface through X,Y-coordinates (0,0).

The picture below schematically represents the surface of water with the position of the wave front from each source of oscillations (M and N) at times t, 2t and 3t, where t is some time interval:

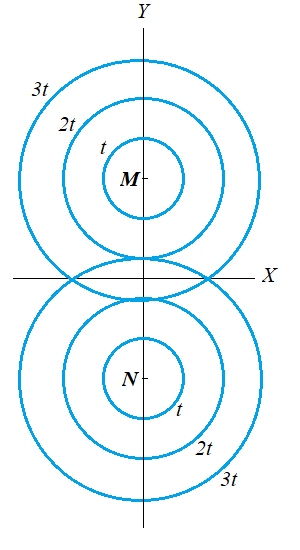
The periodic motion of water molecules, when a wave front and subsequent waves from a single source of oscillations go through them, is, approximately, up and down. Their X and Y coordinates remain, approximately, constant, while Z coordinate oscillates from some maximum positive value A (called amplitude) to negative −A.

This is usually represented as harmonic oscillations in the following form:
z = A·cos(ω·t+φ(r))
where
z is the distance up or down along the Z-axis from the neutral position of a molecule on the XY plane, that is its Z coordinate,
A is the amplitude of these oscillation up and down,
ω is the angular frequency of oscillations of the source that caused the waves on the water surface (number of oscillations per unit of time f multiplied by 2π).
φ(r) is the angular phase shift, which depends on the distance of an oscillating water molecule from the source of oscillations r=√x²+y².

Assume,
T is the time period of the wave (the time one molecule of water takes to go from one highest position all the way down to the lowest point and up to the highest point again),
f is a frequency of oscillations, that is, the number of full oscillation cycles per unit of time,
v is a speed of propagation of the wave front and
λ is the wave length (the distance between two consecutive crests or two consecutive troughs of waves).

Obviously,
v = λ/T because speed is distance divided by time it takes for an object to move along this distance,
f = 1/T because, if one oscillation cycle lasts T units of time, the number of cycles per unit of time is 1/T,
ω = 2π·f = 2π·v/λ because, if one full cycle is equivalent to 2π radians rotation, f rotations per unit of time are equivalent to 2π·f angular rotation per unit of time.

For a water molecule at distance r from the source of oscillations the number of waves between it and the source of oscillations is r/λ (of course, it is not necessarily an integer number). The angular phase shift of oscillations of that molecule relative to the source of oscillation is, therefore, 2π·r/λ.
The absolute phase shift of this molecule's oscillation is then
φ(r) = φ₀ + 2π·r/λ
where φ₀ is the initial phase shift of the source of oscillation at the initial time t=0, which is important to take into consideration when two sources of simultaneous oscillations are not synchronized.

Therefore, we can write an equation that describes the oscillations of a molecule at coordinates (x,y,z) as
z = A·cos(ω·t + φ₀ + 2π·r/λ)

Let's now return to a case with two independent sources of oscillations at points M(0,s,0) and N(0,−s,0) produce waves on the surface of water. For simplicity, assume the frequency of oscillation f (and, therefore, angular frequency ω and wavelength λ) is the same for both sources. Also, assume their amplitudes are the same and equal to A.

For any point P(x,y,0) on a surface of water the distances to both sources of oscillations are: r_M = √x²+(y−s)²
r_N = √x²+(y+s)²

The source of oscillations M produces the oscillations at point P(x,y,0) at time t, according to this formula:
z_M(x,y,t) = A·cos(ω·t +
+ φ_M + 2π·√x²+(y−s)²/λ)
The source of oscillations N produces the oscillations at point P(x,y,0) at time t, according to this formula:
z_N(x,y,t) = A·cos(ω·t +
+ φ_N + 2π·√x²+(y+s)²/λ)

The resulting oscillations at point P(x,y,0) at time t are the combinations of the above two:
z(x,y,t) = z_M(x,y,t) + z_N(x,y,t) =
= A·cos(ω·t+φ_M+2π·r_M/λ) +
+ A·cos(ω·t+φ_N+2π·r_N/λ)

The waves on the water produced by one source of oscillations are simple concentric ones going symmetrically in all directions with the same speed.
The waves from two sources of oscillations, especially with different amplitudes, angular frequencies and initial phase shifts produce a much more complicated picture of interference between two waves.

Just to demonstrate the complexity of the movement of a water molecule responding to two independent sources of oscillations in a more complicated case of different frequencies, amplitudes and phase shifts, here is a graph of periodic oscillations at some point:
z = 0.6·cos(10t+2)+2·cos(2t+6)

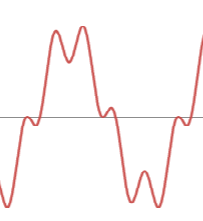
In this case the water molecule at any point will make periodic motion, but within each period the movements up and down will have many intermediate local crests and troughs of different height and depth, so the surface of water will not look like nice concentric waves moving symmetrically from some center, but rather like pretty chaotic set of crests and troughs.

The location of crests and troughs on the water depends very much on two main factors:
(a) the difference in distance from both sources of oscillation and
(b) the wave length.

We will consider only one simple case of wave propagation.
Let's choose the initial time t=0, and assume that both waves produced by both oscillations at their initial position have the same angular frequencies:
ω_M = ω_N = ω,
are at their top amplitude:
φ_M = φ_N = 0,
the same amplitudes:
A_M = A_N = A,
and the distances from these sources to a point on the water surface that we observe are
r₁ and r₂.

If the difference between these distances from sources to a point of observation is a multiple of the wave length, that is
Δr = r₁ − r₂ = N·λ,
where N is an integer number and
λ is the wave length on the water,
the waves coming from two sources of oscillation will be in phase and will enhance each other.
If one wave comes to an observation point at its top crest, another will also come up at its top crest. The result of their superposition will be a crest of a double height.
If one wave comes to an observation point at its bottom trough, another will also come up at its trough. The result of their superposition will be a trough of a double depth.
All intermediate states of the waves will also be in phase and enhance their appearance (double up or double down).

An opposite situation occurs if
Δr = (N+0.5)·λ
In this case two waves are coming to an observation point with opposite amplitudes, which sometimes is called anti-phase.
When a wave from one source of oscillation comes to such a point at its top crest, a wave from another source comes at the bottom trough, and they neutralize each other, the water at such a point will be still.

In other points of observation the waves of two sources will be partially out of phase and the oscillations will make a different picture, not the one as in phase waves, nor as anti-phase, but some mixture of both.

All the oscillations of water can be easily observed. We can see that at one point water goes up and down to a greater extent than in another point because waves of two sources are coming to the first point in phase, while in another point they are in anti-phase.

With this picture of the interference on the water surface in mind let's move to the visible light, taking into consideration that it is transverse oscillations of electromagnetic field, that is electromagnetic waves.

Everything we said about interference of the water waves is applicable to the electromagnetic waves, including such concepts as
amplitude A,
frequency f,
angular frequency ω=2π·f,
period T=1/f,
wavelength λ,
speed of light c=λ/T=λ·f,
angular phase shift φ,
waves being in phase, anti-phase, out of phase.
As another useful formula derived from above definitions, consider this:
ω = 2π·f = 2π/T = 2π·c/λ

Amplitude of the light waves is related to intensity, brightness of light.
Frequency of light, its angular frequency and wavelength are related to the color of light.
So, if different light rays come to the same point (for example, they fall into our eyes or on the flat screen), the interference picture of light of different intensity and color will be visible.

We will consider a simple case of monochromatic light of some wavelength λ, emitted by some source of light, going through two parallel slits, not far from each other, and falling on a screen positioned parallel to slits, as on the picture below that represents a two-dimensional section of this experiment by a plane perpendicular to slits.

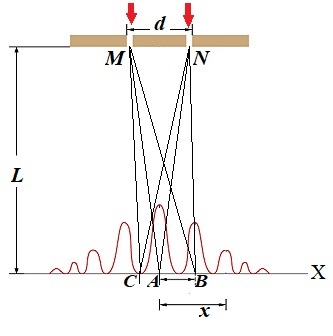
As the flat wave front goes simultaneously through both slits, we can assume that, according to the Huygens principle, two independent sources of light exist at points M and N, sending light rays in all directions, and that electromagnetic waves sent by these slits are of the same wavelength, amplitude and are in phase.

On the screen we will observe certain number of light lines parallel to slits with brightness diminishing as they are further and further from the slits.
The wavy red curve on a picture represents the amplitude (brightness, intensity) of the light on a screen.

We will not see on a screen actual light oscillations (like waves on a water surface) because light oscillations have very high frequency and our eye cannot transmit signals individually to a brain with this frequency. So, the spot on a screen where light oscillates with higher amplitude is visible as a bright spot, and the spot with lower amplitude is visible as dark.
Amplitude is, obviously, a result of interference between rays from our two slits, exactly as on a water surface.

Point A on the screen is on a perpendicular bisector of segment MN and, therefore, is on the same distance from both slits. Therefore, the light rays from both slits M and N, as they reach point A, are in phase and strengthen each other, causing the light line at point A to be the brightest.

For our calculations we will use the distance x on the screen from a middle point A to evaluate the brightness of the light line on a screen.
The other variables are the distance from the slits to our screen L, distance between sources of light d=MN and the wavelength of the light λ.

For any point of observation x on a screen we can calculate the difference Δ between distances S_M and S_N of this point from sources of light M and N to evaluate phase synchronization between two rays coming to this point from these two sources.
S_M = √L²+(x+(d/2))²
S_N = √L²+(x−(d/2))²
Δ = √L²+(x+(d/2))² −
− √L²+(x−(d/2))²

If this difference in distances is equal to an integer number of wavelengths, that is if Δ is equal to n·λ, where n is any integer number, both rays from M and N coming into this point will be in phase, enhance each other and we will have a bright light line on a screen at this point.
If this difference in distances is equal to an integer number of wavelengths plus half of the wavelength, that is if Δ is equal to (n+0.5)·λ, where n is any integer number, both rays from M and N coming into this point will be in anti-phase, weaken each other and we will have a dark line on a screen at this point.

Obviously, there are intermediate values of Δ, which result in gradual change of brightness from maximum, when Δ=n·λ, to minimum, when Δ=(n+0.5)·λ.

In addition, the general strength of light rays from slits M and N will weaken, as the distance of our point of observation to them increases because these rays will fall at higher and higher incident angle to a screen surface.

Finally, we should mention that physicists usually simplify the formulas, using approximation. Let's follow this example and simplify the results above.
We will assume that distance between the slits d=MN is small relative to a distance between slits and a screen L. Also, the distance x from the point of observation and the middle point A also small relative to L.

Let p=√L²+(x+(d/2))² and q=√L²+(x−(d/2))².
Then the following approximate equalities are valid:
Δ = p − q
Multiply and divide this expression by a sum of these two radicals p+q:
Δ = (p² − q²)/(p + q)
p² − q² = 2x·d
p + q ≅ 2√L²+x²

Therefore,
Δ ≅ 2x·d/(2√L²+x²) =
= d·(x/√L²+x²)

But x/√L²+x² is sin(θ), where θ is an incident angle of a ray coming from a midpoint between the slits to a point of observation.

Therefore, if the distance between two sources of light is d and our screen of observation is sufficiently far from the sources of light (L is significantly larger than d), and an incident angle θ of a ray of light falling into an observation point from a midpoint between the slits is such that d·sin(θ) equals to n·λ, where n is any integer number and λ is the wavelength of the light, we will observe the bright light lines.

In a case when d·sin(θ) equals to (n+0.5)·λ we will get a dark lines with graduate change from bright to dark in intermediary points on a screen.

If we use the formula d·sin(θ)=n·λ and determine the possible values of n, we see that
n = d·sin(θ)/λ
Considering sin() cannot be greater than 1 by absolute value, we conclude that |n| is less than d/λ.